Quantum mechanics was invented in the early years of the twentieth century to explain measurements made of very simple systems involving just a very small number of basic particles, like photons, 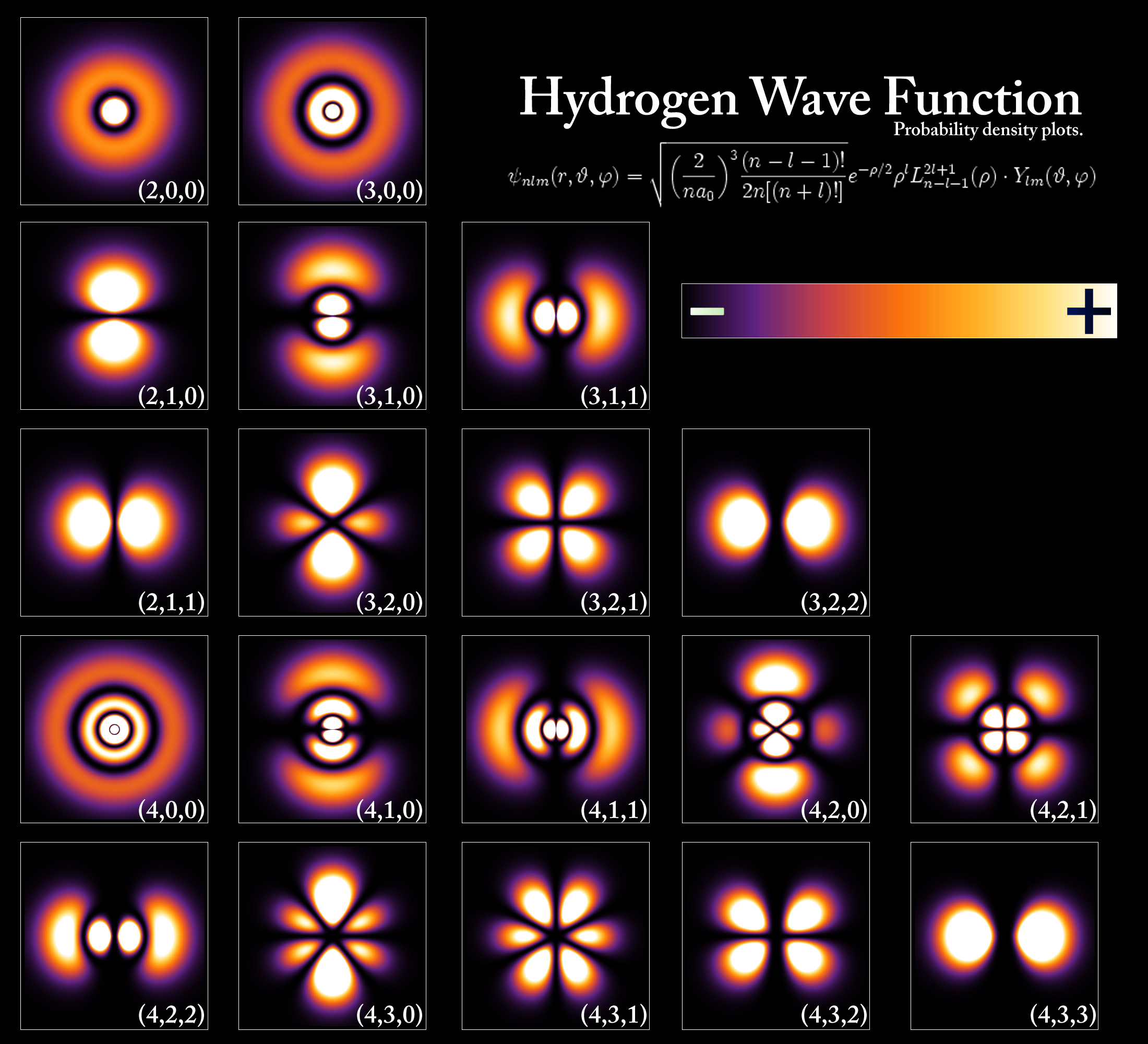atoms or electrons. It was able to make predictions of experiments that could not be explained using Newtonian mechanics. Newtonian mechanics is the theory that explains the macroscopic world, like how a baseball flies, or why the earth circles the sun.

At the heart of quantum theory is the wavefunction, which encapsulates everything that is know about the quantum mechanical system. From the wavefunction it is possible to calculate where each particle is most likely to be, or how fast it’s likely to be moving. These properties cannot be calculated exactly because quantum mechanics tells us that this microscopic world is inherently unpredictable. That is to say that the wavefunction may be prepared in such a way that it is exactly the same each time, but the properties of the system will have some variation in their values each time they are measured.

This naturally leads to the question of just exactly what is the wavefunction. In the early years of quantum theory there was much debate on this subject. This debate was largely silenced in the 1920’s when a group of esteemed physicists, lead by Niels Bohr, came up with a way of thinking about the wavefunction that was accepted by most physicists. They argued that the only thing that physicists are able to do is to make measurements of the system, and that what is happening to the system in between these measurements is unknowable. Furthermore, the wavefunction should only be considered as a mathematical tool to predict the results of future measurements. This viewpoint became known as the Copenhagen Interpretation, and to date is still the conventional view held by most physicists.

Bohr was correct in his interpretation using the paradigms that existed at that time. Measurements were thought to destroy the pre-measurement wavefunction and, which was then recreated in a new form. Indeed, that seems to be the case whenever the system a measurement is made to maximize the precision of the measured value.

In 1988 a new theory was developed that considered measurements that were made with less precision. As you might imagine, the strength of a measurement may be turned down, and as you get down to zero strength the measurement is not being made at all. When a measurement is not made, the wavefunction is not destroyed, and can propagate without changing form. So what happens in between the extremes of a strong measurement and ‘no measurement at all’. This is the regime that has been come to be known as ‘weak measurement’.

With weak measurements, it’s possible to learn something about the wavefunction without completely destroying it. As the measurement becomes very weak, you learn very little about the wavefunction, but leave it largely unchanged. This is the technique that we’ve used in our experiment. We have developed a methodology for measuring the wavefunction directly, by repeating many weak measurements on a group of systems that have been prepared with identical wavefunctions. By repeating the measurements, the knowledge of the wavefunction accumulates to the point where high precision can be restored.

So what does this mean? We hope that the scientific community can now improve upon the Copenhagen Interpretation, and redefine the wavefunction so that it is no longer just a mathematical tool, but rather something that can be directly measured in the laboratory.